What to Review Each Day in Math Grade 6

Everyday Mathematics 2nd Grade Reply Key Unit of measurement 6 Whole Number Operations and Number Stories

Everyday Math Grade 2 Home Link 6.1 Answer Key

Making a Bar Graph

Family unit Note
Your child is exploring different ways to display data. One manner to display data is in a bar graph. For the action below, your child may take to ask a neighbor or phone call a relative to gather the needed pockets data.
Please return this Home Link to school tomorrow.

Question 1.
Pick four people. Count the number of pockets on the clothes that each person is wearing. Record your information in the table.

Answer:

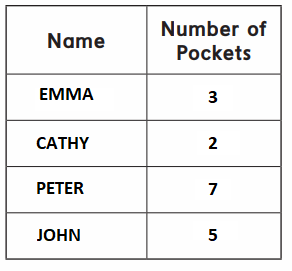
Question 2.
Draw a bar graph for your data. First write each person's proper noun on a line under the graph. Then color the bar above each proper name to show the number of pockets that each person has.

Answer:

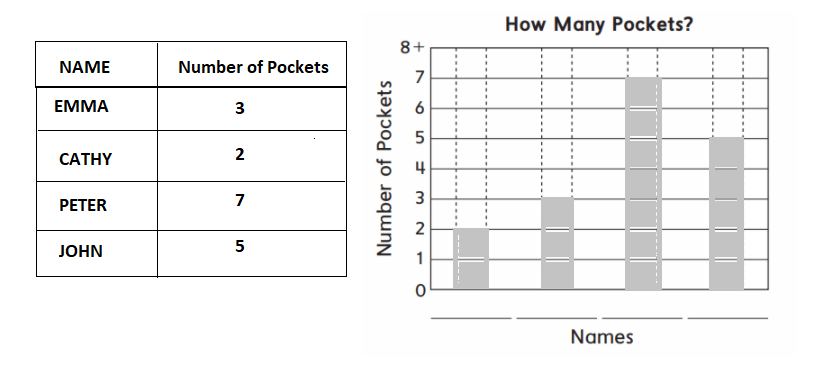
Explanation:
Emma has 3 pockets
Cathy has 2 pockets
Peter has 7 pockets
John has five pockets

Everyday Math Course 2 Dwelling Link vi.2 Answer Cardinal

Comparison Number Stories

For each number story, follow these steps:

• Write the numbers you lot know in the comparison diagram. Employ ? for the number you demand to find.
• Write a number model. Use ? for the number you don't know.
• Solve the problem and answer the question.

Question ane.
Rosa has $29. Omeida has $x. Who has more than coin? __ How much more?
Number model:
Rosa has $ __ more Omeida.

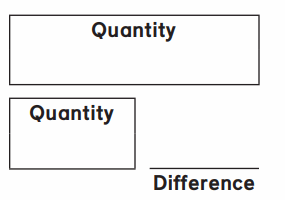
Answer:
Rosa has $19 more then Omeida

Explanation:
Roas has         : $29
Omeida has    : $10

Difference : $29 – $ten =  $19
Rosa has $xix more then Omeida

Question 2.
Omar ran xv miles. Omar ran viii more than miles than Anthony. How many miles did Anthony run?
Number model:
Anthony ran miles.

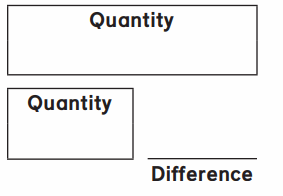
Answer:
Anthony ran  7miles

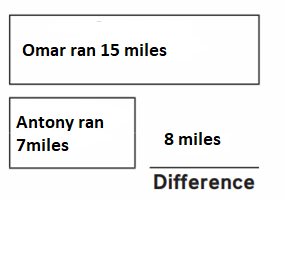
Explanation :
Omar ran xv miles
Omar ran viii more than miles that means:
Anthony ran 15- 8 = 7miles

Everyday Math Grade 2 Home Link 6.3 Respond Key

Add-on and Subtraction Number Stories

Family Note

In today'south lesson your child used diagrams to aid solve addition and subtraction number stories. Diagrams assistance children organize the data from number stories, identify the missing information, and make up one's mind whether to add or decrease to solve the trouble. Organizing data in a diagram too helps children write a number model using ? to represent what they don't know.
Encourage your child to choose a diagram that best matches the way he or she sees the problem. There'southward no right or wrong diagram for a problem. What matters is that it matches the kid'southward thinking.
Please return this Habitation Link to school tomorrow.

Do the post-obit for each number story:
• Write a number model. Use ? to show what y'all need to find. To help, you lot may draw a

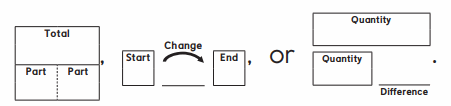
• Solve the problem and write the answer.

Question 1.
Information technology snowed 16 inches in Chicago on Friday night. It snowed 7 inches on Sat night. How much snow did Chicago receive in all?
Number model: __
Answer: __ inches
Answer:
Chicago receive 23 inches in all
Explanation :
No. of inches snowed on Friday night : 16 inches
No. of inches snowed on Saturday dark : 7 inches
Total snowfall received by Chicago : xvi + 7 = 23 inches

Question ii.
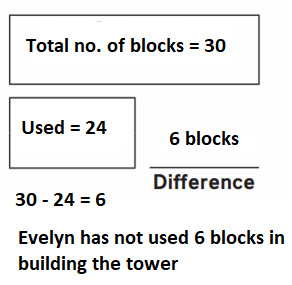
Evelyn has thirty blocks. She used 24 blocks to build a tower.
How many blocks are non used for the tower?
Number model: __
Answer:
Evelyn has not employ 6 blocks in building the tower.

Explanation :
Total no. of blocks Evelyn has : 30
No. of blocks she used in building a tower : 24
No. of blocks not used in building the tower : Full no. of blocks – No. of blocks used in building the tower
: 30 – 24
: 6 blocks
Evelyn has not use 6 blocks in building the tower.

Everyday Math Grade 2 Home Link 6.four Answer Fundamental

Solving Bug

Family unit Note
In form today your child solved addition and subtraction number stories involving the heights and lengths of diverse animals. Some children used mental strategies to solve the stories. Others used tools such as base-x blocks or open number lines. Others drew pictures or state of affairs diagrams to help organize the information from the stories. Delight do not teach your kid a formal method, such every bit the addition method shown at the right. At this phase it is important for children to work with more concrete representations. Children will exist introduced to a formal method for add-on in Lessons vi-7 and vi-8.
Delight return this Home Link to school tomorrow.

Solve the issues below. You may apply base of operations-10 shorthand, open number lines, or any other tool except a calculator to help. You lot may also depict pictures or diagrams.

Question 1.
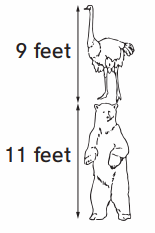
How tall are the ostrich and polar behave together?
Together they are ___ feet tall.

Reply:
twenty feet
Explanation :
Elevation of the bear : eleven feet
Superlative of the ostrich : nine anxiety
Height of ostrich and bear together = Height of the bear + Height of the ostrich
:                                                         = 11 + 9
:                                                         = 20 feet
Height of ostrich and comport together = 20 feet

Question 2.
How much longer is the giant squid than the crocodile?

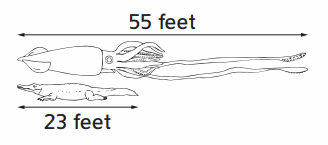
The behemothic squid is ___ feet longer than the crocodile.
Talk to someone virtually how you solved each trouble.
Respond:
The giant squid is 32 anxiety longer than the crocodile.
Caption :
Length of the squid : 55 feet
Length of the crocodile : 23 anxiety
If we subtract the length of the crocodile from the length of the giant squid nosotros will become
55 – 23 = 32 feet
so,
The giant squid is 32 feet longer than the crocodile.

Everyday Math Class 2 Dwelling Link 6.5 Reply Primal

Two-Step Number Stories

Family Note

In today'south lesson your kid solved two-step number stories, which tin exist broken into two parts and then solved in ii steps. For case: Jonathan had vi tickets for rides at the fair. His mother gave him 9 more. So he gave 5 tickets to his friend. How many tickets does he accept at present?
To break this story into 2 parts, ask: What do you know from the story? (Jonathan had 6 tickets.)
What happened first? (He received 9 more than tickets.) What happened next? (He gave away five tickets.)
What do y'all need to detect out? (The number of tickets Jonathan has now.)
The showtime stride is to figure out how many tickets Jonathan had after receiving some from his female parent. The second step is to effigy out how many tickets he had subsequently giving some to his friend. Children are encouraged to solve two-step number stories using a variety of tools: drawings, open number lines, number grids, manipulatives, and diagrams. They too learned to record either one or two number models for each number story—one for each part of the story or ane number model to represent the whole story.

For example: Use one number model, such every bit 6 + 9 – v = ?, for both parts. Or, use 2 number models, such as 6 + 9 = ? and 15 – 5 = ?, i for the beginning role and ane for the 2nd part. Answer: Jonathan now has 10 tickets. Ask your child to explain the steps he or she takes to solve the problem below.
Talk over how his or her number model(s) relates to the number story.
Delight render this Abode Link to school tomorrow.

• Write a number model or number models. Use ? to show the number you need to notice. To help, you may draw a

• Solve the trouble and write the answer.

Question one.
At the embankment, 11 children were playing in the sand. And then vi more children joined them. And then 8 decided to go swimming. How many children were yet playing in the sand?
Number model(s): ___
Answer: __ children
Answer:
9 children were still playing in the sand
Explanation :
Children already playing in the sand : eleven
Children joined them : 6
Full children playing in the sand : eleven + vi = 17
Children decided to go swimming : 8
Children were withal playing in the sand : Full children playing in the sand – Children decided to go swimming
:                                                            : 17 – 8 = 9
9 children were still playing in the sand

Everyday Math Course 2 Home Link half dozen.half dozen Answer Fundamental

Addition Strategies

For each problem:

• Make a ballpark estimate.
• Solve the problem using any strategy you choose. Employ words or pictures to show your thinking.
• Check to make sure your answer makes sense.

Question one.
34 + 59 = ?
Ballpark estimate:
30 + 60 = 90
Strategy:
Combining tens and ones
Answer:
34 + 59 = 93
Explanation :
34 + 59 = ?
Nearest number to 34 is 30 and nearest number to 59 is 60
Then ballpark estimate is 30 + lx = xc
By using the 10's and 'southward strategy
N30 +4] + [l + ix]
At present add together the tens and ones
30 + 50 = eighty
iv + nine = xiii
34 + 59 = 80 + 13 = 93

Question 2.
17 + 68 = ?
Ballpark estimate:
20 + lxx = ninety
Strategy:
Open line strategy
Respond :
17 + 68 = 85
Caption:
The nearest number to 17 is 20
The nearest number to 68 is lxx
So the ballpark will exist twenty + 70 = 90
By using open line strategy we tin calculate 17 + 68
Commencement nosotros have to add 10 to 68
68 + x = 78
And so we take to add 7 to 78
78 + 7 = 85

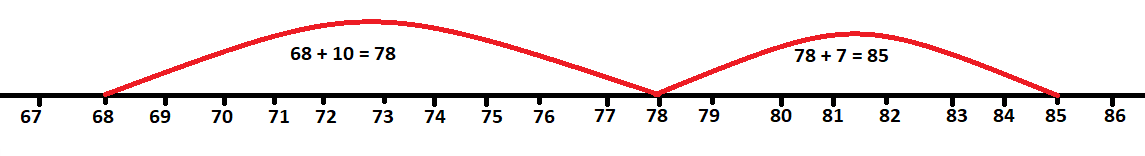
So we get,
17 + 68 = 85

Cull ane of the problems above. Explain your approximate to someone at dwelling. Then explain how you checked to make sure your answer fabricated sense.
Answer :
(As in Question 1)
Caption :
34 + 59 = ?
Nearest number to 34 is thirty and nearest number to 59 is 60
And so, I knew that the ballpark estimate is 30 + 60 = xc
By using the 10's and 's strategy i know
[thirty +four] + [50 + 9]
I have add the tens and ones
30 + fifty = 80
4 + nine = 13
34 + 59 = 80 + 13 = 93
I checked my reply using the calculator equally 34 + 59 = 93
So I know that my answer is correct

Practice

Complete each number sentence to show the expanded course.

Question 3.
__ = 200 + 40 + 6
Answer:
200 + twoscore + 6 = 246
Explanation:
By adding the hundreds, tens and the unit of measurement digits we get :
200 + 40 = 240
240 + 6 = 246
So,
200 + 40 + 6 = 246

Question four.
278 = __+ __ + __
Respond:
200 + 70 + 8 = 278
Caption:
Past splitting the hundreds, tens and the unit digits we get :
200 + 70 + eight = 278

Question 5.
300 + l = __
Respond:
300 + 50 = 350
Explanation:
By adding the hundreds and tens digits we get :
300 + fifty = 350
So,
300  + 50 = 350

Question 6.
420 = __ + __
Answer:
420 = 400 + 20
Explanation:
By splitting the hundreds and tens digits nosotros get :
420 = 400 + 20

Everyday Math Grade 2 Home Link 6.7 Respond Key

Adding with Base of operations-10 Blocks

Family Note

Today children used base-x blocks to help them add numbers. Three types of base-ten blocks were used: A cube represents 1. A long (a rod that is 10 cubes long) represents x. A apartment (a square that is 10 cubes long and x cubes wide) represents 100.
To solve 24 + 32 with base-10 blocks, children first represent each number with blocks or base of operations-ten shorthand:
So children combine the blocks according to blazon (longs with longs; cubes with cubes) and count each type of block: v longs bear witness 5 tens, or fifty; 6 cubes show 6 ones, or 6. The 50 and the six are called partial sums because they are parts of the final sum. Finally, children add the partial sums to find the total: 50 + six = 56.
Children also apply base-ten blocks to add together 3-digit numbers past adding the 100s, 10s, and 1s separately and then combining the partial sums to notice the total.
Please render this Home Link to school tomorrow.

Apply base of operations-ten autograph to show each number. Then write the partial sums and notice the total sum.

Question 1.

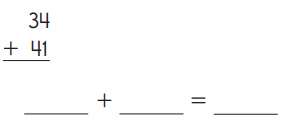
Respond :
34 + 41 = 75
Explanation :

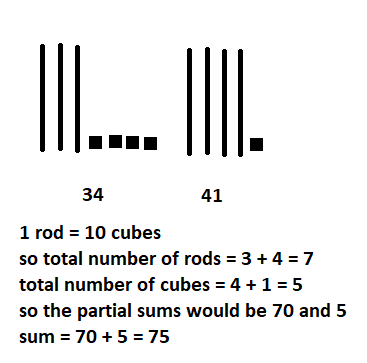
1 rod = 10 cubes
So total number of rods = iii + 4 = 7
Total number of cubes = 4 + ane = 5
Partial sums are 70 and five
Sum = 70 + v = 75

Question 2.

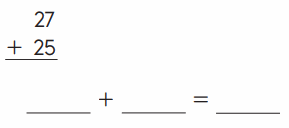
Answer:
27 + 25 = 52
Caption :

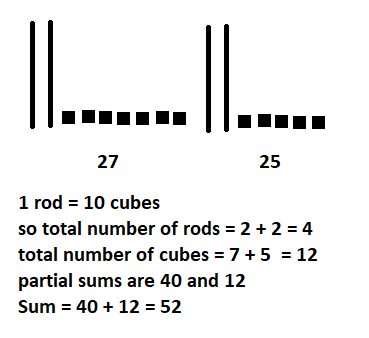
1 rod = 10 cubes
So full number of rods = 2 + 2 = 4
Full number of cubes = vii + 5  = 12
Partial sums are 40 and 12
Sum = forty + 12 = 52

Explain to someone at habitation how you use base-10 blocks to add together.

Exercise

Complete each number sentence to bear witness the expanded course of a number.

Question iii.
__ = 500 + 30 + 2
Respond:
500 + 30 + 2 = 532
Caption :
By adding the hundreds, tens and the unit of measurement digits we get :
500 + xxx = 530
530 + two = 532
So,
500 + 30 + two = 532

Question iv.
340 = __ + __
Answer:
340 = 300 + 40
Explanation :
By splitting the number we get the ii partial sums
300 and 40
So
340 = 300 + 40

Question five.
400 + v = __
Respond:
400 + v = 405
Explanation :
Past calculation the 2 partial sums we get :
400 + five = 405

Question 6.
609 = __ + __
Respond:
609 = 600 + ix
Explanation :
By splitting the number into its partial sums we get
609 = 600 + 9

Everyday Math Grade 2 Home Link 6.8 Answer Key

More than Partial Sums

Family Annotation

In the previous lesson your child used base-x blocks to help discover partial sums. Today your child used expanded form. Expanded form shows numbers broken apart into a sum of place-value pieces, such as hundreds, tens, and ones. For example, the expanded course for 324 is 300 + 20 + iv.
To solve 324 + 255, your child can first write or think about each number in expanded form, then use the expanded form to assistance discover the partial sums:

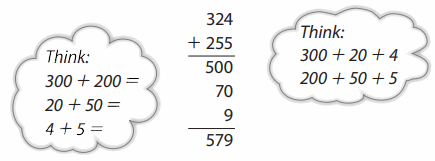
Encourage your child to use place-value language when working with this method. For instance, when calculation the 100s in this example, guide your kid to say "300 + 200 = 500," not "3 + 2 = 5." Writing the expanded course can help children call back to use the correct language.
This method of finding fractional sums and and then combining the fractional sums to notice the total is called partial-sums improver. Partial-sums addition was introduced merely recently, so permit plenty of time for practice before expecting your kid to use it easily.
Please return this Home Link to school tomorrow.

Make full in the unit box. For each problem:

• Make a ballpark judge. Solve the trouble using partial-sums addition. Show your work.
• Utilize your ballpark estimate to check if your respond makes sense.

Question 1.

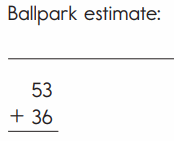
Answer:
Ballpark Judge :
l + 30 = 80
Reply : 53 + 36 = 89
Caption :
According To Ballpark Estimate :
l + xxx = 80
Add together the tens digits:
l + thirty = lxxx
Now add the units digits :
three + 6 = 9
Now add them together
80 + ix = 89
53 + 36 = 89

Question ii.

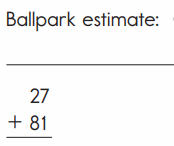
Answer:
According To Ballpark Guess :
thirty + 80 = 110
Answer :
27 + 81 = 108
Explanation :
Ballpark Estimate : 30 + 80 = 110
Add the 10s digits
xx + 80 = 100
7 + 1 = 8
100 + 8 = 108 so we go
27 + 81 = 108

Question 3.

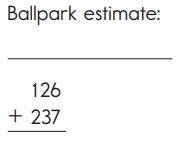
Answer:
Ballpark estimate :
Answer :
125 + 240 = 365
Explanation :
Ballpark estimate : 125 + 240 = 365
Now add the hundreds
100 + 200 =  300
At present add the tens
20 + 30 = 50
At present add the ones
6 + 7 = xiii
Now add the hundreds, tens and ones
300 + 50 + 13 = 363
And so we go,
126 + 237 = 363

Everyday Math Grade 2 Habitation Link 6.nine Reply Fundamental

Subtraction Number Stories

Family unit Notation

In today'southward lesson, your child solved subtraction number stories using different tools and strategies based on place-value concepts and explained his or her thinking in drawings and words. Existence able to solve issues in multiple ways and explain their strategies helps children become flexible problem solvers.
Every bit your child solves these problems, ask him or her to explain the strategy.
Please return this Home Link to school tomorrow.

Question 1.
Sam is on a baseball team. This twelvemonth he set a goal of scoring 36 runs for his team. So far Sam has scored 26 runs. How many more runs does Sam need to score in lodge to meet his goal?
__ runs
Reply:
ten runs
Explanation :
Full runs to be scored : 36
Runs Sam scored : 26
Runs need for Sam to attain is goal : 36 – 26 = 10 runs
10 runs demand more than to score to run across his goal

Question 2.
Sam helped his mother unload the dishwasher. As he was putting the silverware abroad, Sam counted 21 spoons and 13 forks. How many more spoons than forks did Sam unload?
__ spoons
Answer:
viii spoons
No. of spoons : 21 spoons
No. of forks : xiii forks
21 – 13 = 8
8 spoons more than then forks

Practice

Question 3.
a.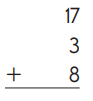
Answer:
28
Explanation :
17 + 3 + 8 = 28

b.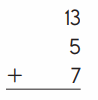
Answer:
25
Explanation :
13 + 5 + 7 = 25

c.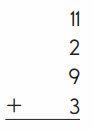
Reply:
25
Explanation :
11 + ii + 9 + 3 = 25

d.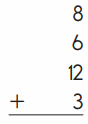
Answer:
29
Explanation :
viii + vi + 12 + three = 29

Everyday Math Grade 2 Dwelling house Link 6.10 Answer Key

How Many?

Family unit Annotation

Your kid has been working with arrays to develop readiness for multiplication. Arrays are rectangular arrangements of objects that have the same number of objects in each row. For example, a 3-past-5 array is shown at the right.
Your kid found the total number of objects in each array and learned to write addition number models to represent arrays. One case of an addition number model for this assortment is 5 + 5 + 5 = fifteen. At that place are 15 Xs in all.
When your kid writes an addition number model to show the number of objects in a v-by-4 array, he or she is building understanding of the meaning of iv 5s, or 4 × 5.
Delight return this Domicile Link to school tomorrow.

Question i.
Draw an array with 2 rows of Xs with 8 Xs in each row.
Write an addition number model for the array.
Answer:

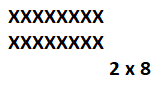
Number model :
8 + 8 = 16

Question 2.
Depict an array with four rows of Xs with 6 Xs in each row.
Write an addition number model for the array.
Respond:

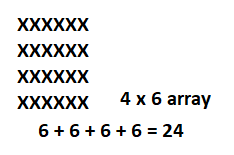
Number model :
6 + 6 + six + 6 = 24

Question 3.
Draw an array with 3 rows of Xs with 7 Xs in each row.
Write an addition number model for the assortment.
Answer:

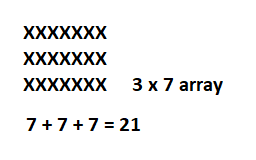
Number model :
7 + 7 + 7 = 21

colemanandust.blogspot.com

Source: https://ccssmathanswers.com/everyday-math-grade-2-answers-unit-6/

Share this post